There’s a problem with the D Plane that renders it almost useless for most golfers. Professor Jergensen highlighted the issue himself; the D Plane is only applicable for “perfect” strikes, where the ball is hit with the clubface’s sweet spot. This is something few golfers achieve, let alone consistently.

When the ball isn’t struck with the clubface’s sweet spot, there are other forces at play that determine the ball’s flight.(1) This chapter is not about that failing. I believe the D Plane concept, although more involved than the two-dimensional “Ball Flight Laws”, is still an overly simplified model of what actually occurs during impact.

There are two main problems with the D Plane. The first is when exactly, during the dynamic impact between club and ball, the D Plane is measured.

Professor Jorgensen, when first introducing the D Plane states: “Euclid, the famous geometer, say something to the effect that two intersecting lines determine a plane. The normal to the clubface and the line along which the clubhead is moving at impact intersect at the ball and therefore determine a plane.”
It’s true that the normal to the clubface and the clubhead path intersect at the ball, but they do so for a period of time, namely around 0.0005 seconds, according to Professor Jorgensen’s own book.

Although those 0.0005 seconds of impact aren’t long at all, at lot happens during that time. The clubface comes into contact with the ball and compresses it. The ball “sticks” to the clubface and then separates from it before flying away.

During this impact interval, the ball and clubface are both moving. So when exactly is the D Plane measured? If we turn to the “Technical Appendix - Section 3” in The Physics of Golf, Professor Jorgensen goes into further detail on the D Plane. Here he explains: “When a golfer swings a club, he is preparing for a collision between the clubhead of equivalent mass M having a velocity V and a stationary ball of mass m.... ...Just before the collision, the orientation of the clubhead may be specified by a vector N, the normal to the face of the club, at an angle θ to the velocity vector. The two vectors V and N define a plane, which will be called the D Plane, since it is descriptive of the collision.”

Professor Jorgensen goes on to say: “Just after the collision the clubhead will be moving with a velocity W, smaller than V, but essentially in the same direction as V.”

So here Professor Jorgensen explains the D Plane is created by the vectors “V” which is the clubhead path (and it’s speed) before impact and the normal to the clubface “N”, which again is established “just before the collision”. Surprisingly this means the D Plane’s point of origin is established before the clubface even comes into contact with the ball. How much before the ball we cannot say, only that it’s “just before”.

The two vectors creating the D Plane “just before” impact then point in different directions when the clubface first touches the ball. They change in direction as the ball and clubface remain touching, and are pointing in different directions when the ball finally separates from the clubface. In my opinion, the Descriptive Plane is a misnomer. It doesn’t describe impact. It describes two vectors “just before” impact, and also ignores the fact those two vectors during impact are in a state of flux.

The second problem I have with the D Plane concept is how the vector for the clubhead path is established. I have made the assumption, like everyone else seems to, that this vector is the tangent of the circular clubhead path.

Professor Jorgensen doesn’t state this vector is a tangent (or secant) of the clubhead path, it simply is the clubhead path. Professor Jorgensen treats the clubhead path as a straight line, not only in diagrams but when explaining how to manipulate the D Plane. He states in chapter “The D Plane as a Practical Tool”:

“It is suggested that a golfer take a five iron in hand as he reads this description of the use of the D Plane. If the reader takes the usual stance with a five iron and swings the club directly in the direction of an assumed target with the clubface square to the target, neither toed in or toed out, then the D Plane for the swing will be a vertical plane containing the velocity of the clubhead, the velocity vectors of the ball and the normal to the clubface, and the target.”

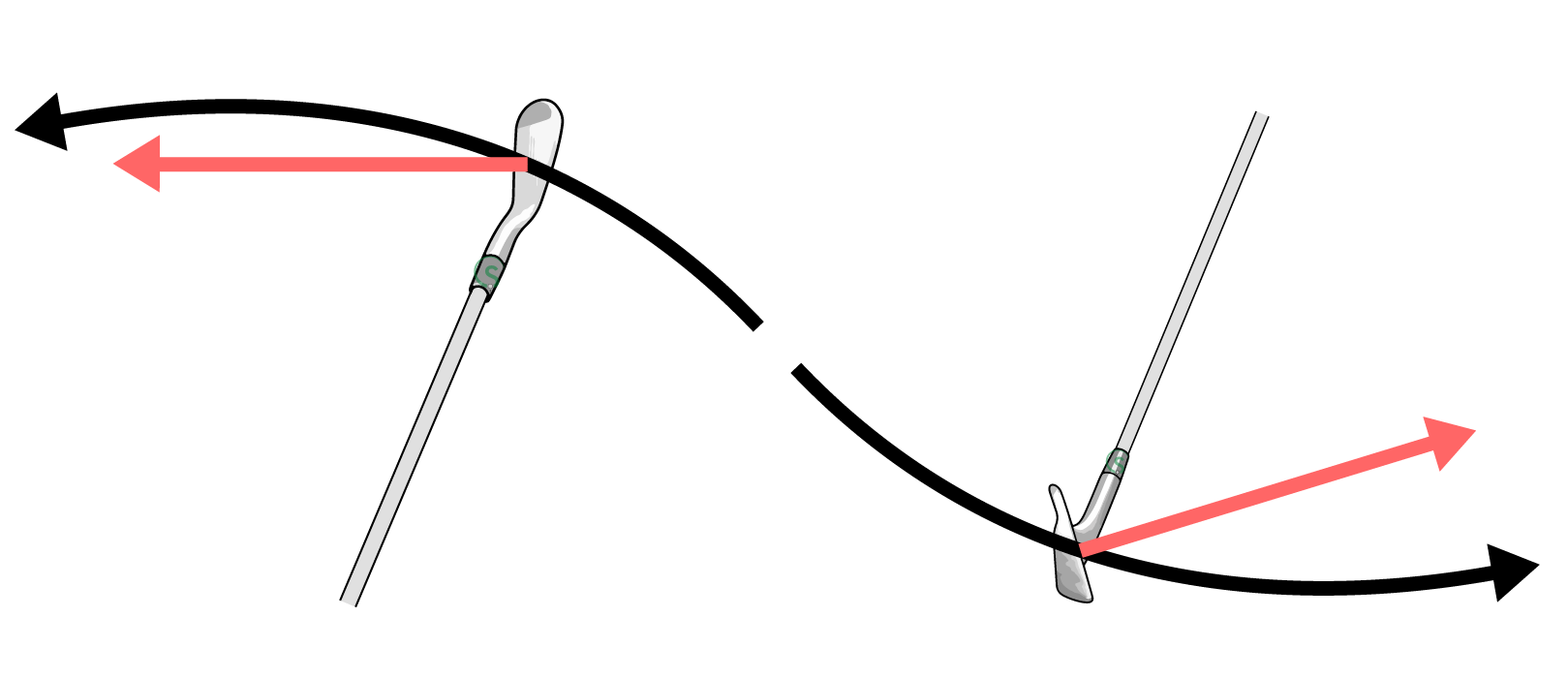
It would be next to impossible to swing the club in the manner suggested by Professor Jorgensen. To swing the club "directly in the direction of an assumed target" would be to swing the clubhead in a straight line. The clubhead moves in a circular fashion around the body.

The D Plane, established using the vector of the clubhead path would actually look like pictures above. Here you can see the two-dimensional D Plane becomes three-dimensional, and wouldn't describe the ball flight vectors.

In summary: besides only applying to “perfectly hit” impacts, the D Plane concept ignores its vectors move during the impact interval, measures them at an undetermined time before impact, and assumes the clubhead path is a straight line.